2.
In cyclic quadrilaterla ABCD, AB=2, BC=3, CD=10, and DA=6.
Let P be the intersection of lines AB and CD.
Find the length BP.
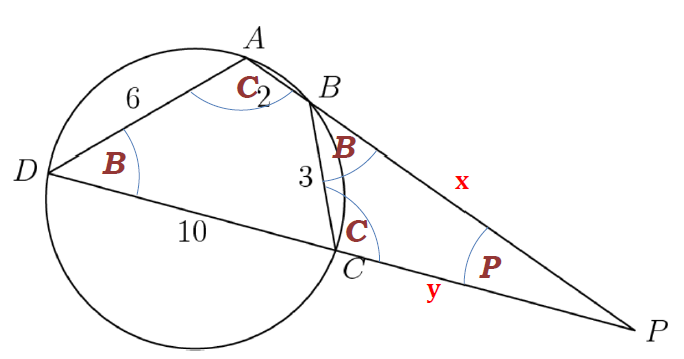
\(\text{Let $BP =x$} \\ \text{Let $PA =2+x$} \\ \text{Let $PC =y$} \\ \text{Let $PD =10+y$} \)
\(\text{Let $\angle BPC = P $} \\ \text{Let $\angle CBP = B $} \\ \text{Let $\angle ABC = 180^\circ -B $} \\ \text{Let $\angle ADC = 180^\circ - \angle ABC =180^\circ-(180^\circ -B)=B $} \)
1.
Intersecting secants theorem:
see: https://en.wikipedia.org/wiki/Intersecting_secants_theorem
\(\begin{array}{|rcll|} \hline \mathbf{BP\cdot PA} &\mathbf{=}& \mathbf{PC\cdot PD} \quad | \quad \text{Intersecting secants theorem} \\\\ x\cdot (2+x) &=& y\cdot (10+y) \\ \hline \end{array} \)
2.
sin-theorem:
\(\begin{array}{|lrcll|} \hline 1. & \dfrac{\sin(P)}{3} &=& \dfrac{\sin(B)}{y} \\ & \dfrac{\sin(P)}{\sin(B)} &=& \dfrac{3}{y} \\\\ 2. & \dfrac{\sin(P)}{6} &=& \dfrac{\sin(B)}{2+x} \\ & \dfrac{\sin(P)}{\sin(B)} &=& \dfrac{6}{2+x} \\ \hline & \dfrac{\sin(P)}{\sin(B)} = \dfrac{3}{y} &=& \dfrac{6}{2+x} \\\\ & \dfrac{3}{y} &=& \dfrac{6}{2+x} \\\\ & \dfrac{y}{3} &=& \dfrac{2+x}{6} \\\\ & y &=& \dfrac{3}{6}\cdot(2+x) \\\\ & \mathbf{y} & \mathbf{=}& \mathbf{\dfrac{1}{2}\cdot(2+x)} \\\\ & x\cdot (2+x) &=& y\cdot (10+y) \quad | \quad y = \dfrac{1}{2}\cdot(2+x) \\\\ & x\cdot (2+x) &=& \dfrac{1}{2}\cdot(2+x)\cdot \left(10+\dfrac{1}{2}\cdot(2+x)\right) \\\\ & x &=& \dfrac{1}{2} \cdot \left(10+ \dfrac{1}{2}\cdot(2+x) \right) \quad | \quad \cdot 2 \\\\ & 2x &=& 10+ \dfrac{1}{2}\cdot(2+x) \quad | \quad \cdot 2 \\\\ & 4x &=& 20+ 2+x \\\\ & 3x &=& 22 \\\\ & x &=& \dfrac{22}{3} \\\\ & \mathbf{ x } & \mathbf{=} & \mathbf{7.\bar{3}} \\ \hline \end{array}\)
![]()
There are many ways to circumscribe a rectangle R about a 5 x 10 rectangle so
that each vertex of the 5 x 10 rectangle is on a different side of R.
Rectangle R's area is 110. what is the maximum area of a rectangle R
that can be circumscribed about a 5 x 10 rectangle?
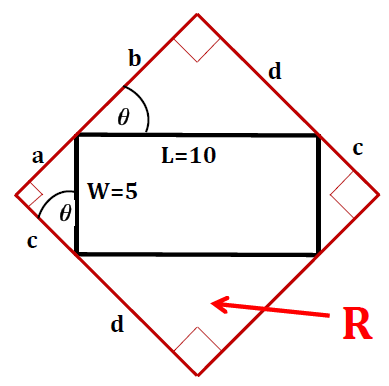
Let:
\(\begin{array}{|rcll|} \hline a &=& W\sin(\theta) \\ c &=& W\cos(\theta) \\ \hline \end{array} \begin{array}{|rcll|} \hline d &=& L\sin(\theta) \\ b &=& L\cos(\theta) \\ \hline \end{array} \)
The area A of the circumscribing rectangle:
\(\begin{array}{|rcll|} \hline \mathbf{A(\theta)} &\mathbf{=}& \mathbf{(a+b)(c+d)} \\\\ A(\theta) &=& \Big( W\sin(\theta)+L\cos(\theta) \Big) \Big( W\cos(\theta)+L\sin(\theta) \Big) \\ &=& W^2\sin(\theta)\cos(\theta) +L\cdot W\sin^2(\theta) + LW\cos^2(\theta) + L^2\sin(\theta)\cos(\theta) \\ &=& (W^2+L^2)\sin(\theta)\cos(\theta) +L\cdot W\Big((\sin^2(\theta) + \cos^2(\theta)\Big) \quad | \quad \sin^2(\theta) + \cos^2(\theta) = 1 \\ &=& (W^2+L^2)\sin(\theta)\cos(\theta) +L\cdot W \quad | \quad \sin(\theta)\cos(\theta) = \dfrac{\sin(2\theta)}{2} \\ &=& (W^2+L^2)\dfrac{\sin(2\theta)}{2} +L\cdot W \\ && \color{red}\underline{\text{maximize}}:\\ && \qquad \color{red}\sin(2\theta) = 1 \\ && \qquad \color{red}2\theta = \arcsin(1) \\ && \qquad \color{red} 2\theta = 90^\circ \\ && \qquad \color{red} \theta = 45^\circ \\ A_{\text{max}}(45^\circ) &=& (W^2+L^2)\dfrac{\sin(2\cdot 45^\circ)}{2} +L\cdot W \\ &=& (W^2+L^2)\dfrac{\sin(90^\circ)}{2} +L\cdot W \quad | \quad \sin(90^\circ) = 1 \\ &=& \dfrac{1}{2} (W^2+L^2) +L\cdot W \quad | \quad \sin(90^\circ) = 1 \\ &=& \dfrac{1}{2} (W^2+L^2+2LW) \\ &=& \dfrac{1}{2} (W+L)^2 \\ \hline \end{array}\)
The maximum area:
\(\begin{array}{|rcll|} \hline A_{\text{max}} &=& \dfrac{1}{2} (W+L)^2 \\\\ &=& \dfrac{1}{2} (5+10)^2 \\\\ &=& \dfrac{15^2}{2} \\\\ &=& \dfrac{225}{2} \\\\ &=& 112.5 \\ \hline \end{array}\)
The maximum area is 112.5
source: https://www.youtube.com/watch?v=q3ZnOvhEJWo
![]()