heureka

I got 32 when I tried to solve this but it was incorrect.. ):

\(\text{The function $h(x)$ is defined as: $h(x) = \left\{ \begin{array}{cl} \lfloor 4x \rfloor & \text{if } x \le \pi, \\ 3-x & \text{if }\pi < x \le 5.2, \\ x^2& \text{if }5.2< x. \end{array}\right. $}\\ \text{Find $h\Big(h\left(\sqrt{2} \right) \Big)$.} \)

\(\begin{array}{|rcll|} \hline h\left(\sqrt{2} \right) &=& \lfloor 4\cdot \sqrt{2} \rfloor \\ &=& \lfloor 4\cdot 1.41421356237 \rfloor \\ &=& \lfloor 5.65685424949 \rfloor \\ &=& 5 \\\\ h(5) &=& 3-5 \\ &=& -2 \\\\ \mathbf{h\Big(h\left(\sqrt{2} \right) \Big)} & =& \mathbf{ -2 } \\ \hline \end{array} \)

Thank you, CPhill !

Evaluate the sum

\(\large{s = 1 + \dfrac{3}{3} + \dfrac{5}{9} + \dfrac{7}{27} + \dfrac{9}{81} + \dotsb }\) 

Determine the value of

\(\large \dfrac{\dfrac{2016}{1} + \dfrac{2015}{2} + \dfrac{2014}{3} + \dots + \dfrac{1}{2016}}{\dfrac{1}{2} + \dfrac{1}{3} + \dfrac{1}{4} + \dots + \dfrac{1}{2017}}.\)

\(\begin{array}{|rcll|} \hline && \mathbf{\dfrac{\dfrac{2016}{1} + \dfrac{2015}{2} + \dfrac{2014}{3} + \dots + \dfrac{1}{2016}}{\dfrac{1}{2} + \dfrac{1}{3} + \dfrac{1}{4} + \dots + \dfrac{1}{2017}} } \\\\ &=& \dfrac{\dfrac{2017-1}{1} + \dfrac{2017-2}{2} + \dfrac{2017-3}{3} + \dots + \dfrac{2017-2016}{2016}} {\dfrac{1}{2} + \dfrac{1}{3} + \dfrac{1}{4} + \dots + \dfrac{1}{2016}+ \dfrac{1}{2017}} \\\\ &=& \dfrac{\dfrac{2017}{1} + \dfrac{2017}{2} + \dfrac{2017}{3} + \dots + \dfrac{2017}{2016}-2016\cdot 1} {\dfrac{1}{2} + \dfrac{1}{3} + \dfrac{1}{4} + \dots + \dfrac{1}{2016}+ \dfrac{1}{2017}} \\\\ &=& \dfrac{\dfrac{2017}{2} + \dfrac{2017}{3} + \dots + \dfrac{2017}{2016}+2017-2016 } {\dfrac{1}{2} + \dfrac{1}{3} + \dfrac{1}{4} + \dots + \dfrac{1}{2016}+ \dfrac{1}{2017}} \\\\ &=& \dfrac{\dfrac{2017}{2} + \dfrac{2017}{3} + \dots + \dfrac{2017}{2016}+1 } {\dfrac{1}{2} + \dfrac{1}{3} + \dfrac{1}{4} + \dots + \dfrac{1}{2016}+ \dfrac{1}{2017}} \\\\ &=& \dfrac{\dfrac{2017}{2} + \dfrac{2017}{3} + \dots + \dfrac{2017}{2016} +1 } {\left( \dfrac{1}{2} + \dfrac{1}{3} + \dfrac{1}{4} + \dots + \dfrac{1}{2016}+\dfrac{1}{2017} \right)\times \dfrac{2017}{2017} } \\\\ &=& \dfrac{\dfrac{2017}{2} + \dfrac{2017}{3} + \dots + \dfrac{2017}{2016} +1 } {\left( \dfrac{2017}{2} + \dfrac{2017}{3} + \dfrac{2017}{4} + \dots + \dfrac{2017}{2016}+\dfrac{2017}{2017} \right)\times \dfrac{1}{2017} } \\\\ &=& \dfrac{\dfrac{2017}{2} + \dfrac{2017}{3} + \dots + \dfrac{2017}{2016} +1 } {\left( \dfrac{2017}{2} + \dfrac{2017}{3} + \dfrac{2017}{4} + \dots + \dfrac{2017}{2016}+1 \right)\times \dfrac{1}{2017} } \\\\ &=& \dfrac{\left(\dfrac{2017}{2} + \dfrac{2017}{3}+ \dfrac{2017}{4} + \dots + \dfrac{2017}{2016} +1\right) } {\left( \dfrac{2017}{2} + \dfrac{2017}{3} + \dfrac{2017}{4} + \dots + \dfrac{2017}{2016}+1 \right) } \times 2017 \\\\ &=& \mathbf{2017} \\ \hline \end{array}\)

Thank you, CPhill !

Consider...

\(\bar{r} = 2-i\\ r = 2+i\\ \bar{r}z+r\bar{z}=20 \quad | \quad \bar{r}z=r\bar{z}\\ 2\bar{r}z = 20 \\ \bar{r}z = 10 \\ (2-i)z = 10\\ 2z-\underbrace{iz}_{=0} = 20 \\ 2z=10\\ \mathbf{z=5}\)

Lines with slopes -1 and -2 are drawn through the first quadrant point (a,b) forming one triangle

with a side on the x-axis and the other with one side on the y-axis.

What is the total area of the two shaded triangles? (Write you answers in terms of a and b)

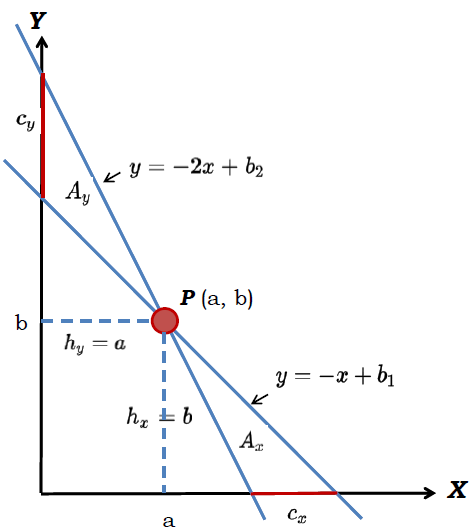
\(\mathbf{b_1=\ ?}\)

\(\begin{array}{|rcll|} \hline y&=&-x+b_1 \quad | \quad P(a,b) \text{ on line} \\ b&=&-a+b_1 \\ \mathbf{b_1} &=& \mathbf{a+b} \\\\ \mathbf{y} &=& \mathbf{-x+(a+b)} \\ \hline \end{array}\)

\(\mathbf{b_2=\ ?}\)

\(\begin{array}{|rcll|} \hline y&=&-2x+b_2 \quad | \quad P(a,b) \text{ on line} \\ b&=&-2a+b_2 \\ \mathbf{b_2} &=& \mathbf{2a+b} \\\\ \mathbf{y} &=& \mathbf{-2x+(2a+b)} \\ \hline \end{array}\)

\(\mathbf{y=0}\\ \mathbf{c_x=\ ?} \)

\(\begin{array}{|rcll|} \hline 0 &=& -x_1+(a+b) \\ \mathbf{x_1} &=& \mathbf{a+b} \\\\ 0 &=& -2x+(2a+b) \\ 2x &=& 2a+b \\ \mathbf{x_2} &=& \mathbf{a+\dfrac{b}{2}} \\\\ c_x &=& x_1-x_2 \\ &=& a+b - \left(a+\dfrac{b}{2} \right) \\ \mathbf{c_x} &=& \mathbf{\dfrac{b}{2}} \\ \hline \end{array}\)

\(\mathbf{x=0}\\ \mathbf{c_y=\ ?}\)

\(\begin{array}{|rcll|} \hline y_1 &=& -0 +(a+b) \\ \mathbf{y_1} &=& \mathbf{a+b} \\\\ y_2 &=& -2\cdot 0+(2a+b) \\ &=& 2a+b \\ \mathbf{y_2} &=& \mathbf{2a+b} \\\\ c_y &=& y_2-y_1 \\ &=& 2a+b-(a+b) \\ \mathbf{c_y} &=& \mathbf{a} \\ \hline \end{array}\)

\(\mathbf{A_x=\ ?} \)

\(\begin{array}{|rcll|} \hline A_x &=& \dfrac{c_xh_x}{2} \quad | \quad h_x = b,\qquad c_x = \dfrac{b}{2} \\\\ &=& \dfrac{\dfrac{b}{2}\cdot b}{2} \\\\ \mathbf{A_x} &=& \mathbf{\dfrac{b^2}{4}} \\ \hline \end{array}\)

\(\mathbf{A_y=\ ?}\)

\(\begin{array}{|rcll|} \hline A_y &=& \dfrac{c_yh_y}{2} \quad | \quad h_y = a,\qquad c_y = a \\\\ &=& \dfrac{a\cdot a}{2} \\\\ \mathbf{A_y} &=& \mathbf{\dfrac{a^2}{2}} \\ \hline \end{array}\)

\(\mathbf{A=\ ?}\)

\(\begin{array}{|rcll|} \hline A&=& A_x + A_y \\ &=& \dfrac{b^2}{4} + \dfrac{a^2}{2} \\ \mathbf{A} &=& \mathbf{\dfrac{1}{2}\cdot \left( a^2 + \dfrac{b^2}{2} \right) } \\ \hline \end{array} \)

Prove this identity.

\(\large \dfrac{1+\sin(\theta)}{1-\sin(\theta)}=\dfrac{\csc(\theta)+1}{\csc(\theta)-1}\)

\(\begin{array}{|rcll|} \hline && \dfrac{1+\sin(\theta)}{1-\sin(\theta)} \\\\ &=& \dfrac{1+\sin(\theta)}{1-\sin(\theta)}\cdot \dfrac{ \dfrac{1}{\sin(\theta)} } {\dfrac{1}{\sin(\theta)}} \\\\ &=& \dfrac{ \Big(1+\sin(\theta)\Big) \dfrac{1}{\sin(\theta)} } {\Big(1-\sin(\theta) \Big)\dfrac{1}{\sin(\theta)}} \\\\ &=& \dfrac{ \dfrac{1}{\sin(\theta)} + \dfrac{\sin(\theta)} {\sin(\theta)} } { \dfrac{1}{\sin(\theta)} - \dfrac{\sin(\theta)}{\sin(\theta)} } \\\\ &=& \dfrac{ \dfrac{1}{\sin(\theta)} + 1 } { \dfrac{1}{\sin(\theta)} - 1 } \quad | \quad \boxed{\dfrac{1}{\sin(\theta)} = \csc(\theta) } \\\\ &=& \dfrac{ \csc(\theta) + 1 } { \csc(\theta) - 1 } \\ \hline \end{array}\)

A four-digit hexadecimal integer is written on a napkin such that the units digit is illegible.

The first three digits are 4, A, and 7.

If the integer is a multiple of 17 base ten, 

what is the units digit?

\(\begin{array}{|rcll|} \hline \mathbf{4A7x_{16}} &=& \mathbf{ ( ( 4\cdot 16+A )\cdot 16+7)\cdot16+x } \quad | \quad A = 10 \\ &=& ((4\cdot 16+10)\cdot 16+7)\cdot16+x \\ &=& (74\cdot 16+7)\cdot16+x \\ &=& 1191\cdot16+x \\ &=& \mathbf{19056 +x},\qquad x=0,1,\ldots, 15 \\ \hline \end{array} \)

\(\begin{array}{|rcll|} \hline \mathbf{19056 +x} &=& \mathbf{n\cdot 17},\ n \in \mathbf{Z} \\\\ 19056 +x &\equiv& 0 \pmod {17} \quad | \quad 19056 \pmod {17} = 16 \\ 16 +x &\equiv& 0 \pmod {17} \\ 16 +x &=& n\cdot 17 \\ x &=& n\cdot 17 -16 \quad | \quad n = 1 \\ x &=& 1\cdot 17 -16 \\ \mathbf{x} &=& \mathbf{1} \\ \hline \end{array}\)

What is the least positive integer value of \(x\) such that \((2x)^2 + 2\cdot 37\cdot 2x + 37^2\) is a multiple of \(47\)?

\(\begin{array}{|rcll|} \hline \mathbf{(2x)^2 + 2\cdot 37\cdot 2x + 37^2} &=& \mathbf{\left(2x+37\right)^2} \\\\ \left(2x+37\right)^2 &=& \left(47n\right)^2 \\ 2x+37 &=& 47n,\ \qquad n \in \mathbf{Z} \\ 2x &=& 47n - 37 \\\\ \mathbf{ x } &=& \mathbf{\dfrac{ 47n - 37 } {2}} \quad | \quad 47n - 37 \text{ is even, so } n \text{ is odd} \\\\ \mathbf{ x_{\text{least positive integer}} } &=& \mathbf{\dfrac{ 47n - 37 } {2}},\ \text{if } n = 1 \\\\ &=& \dfrac{ 47 - 37 } {2} \\\\ &=& \dfrac{ 10 } {2} \\\\ \mathbf{ x_{\text{least positive integer}} } &=& \mathbf{5} \\ \hline \end{array}\)