Melody

\((x^2 + 2x + 5 - x - 4)(3x^2 - x - 4 + 2x + 8) \ge 0\\ (x^2 + x + 1)(3x^2 + x +4) \ge 0\\ \qquad \text{consider the roots of}\; x^2+x+1\\ \qquad \triangle=b^2-4ac=1-4=-3<0 \qquad\text{ no real roots}\\ \qquad \text{consider the roots of}\; 3x^2+x+4\\ \qquad \triangle=b^2-4ac=1-48=-47<0 \qquad\text{ no real roots}\\ \)

Since the coefficiant of x^4 is positive, and there are not real roots. 

this funtion will always be positve.

x is in the set of real numbers (no restrictions) 

ok

triangle LMN is congruent to triangle ONM  so LM=NO

And NP is a half of NO

And RQ=NP

So the horizontal distance from Q to L is 3 times the horizontal distance from Q to R

Triangles  RPQ and LPQ share the same base, that is PQ

But the perpendicular height of LPQ is three times that of RPQ 

Since the area of triangle RPQ is  27,

It follows that the area of LPQ is  3*27=  81u^2

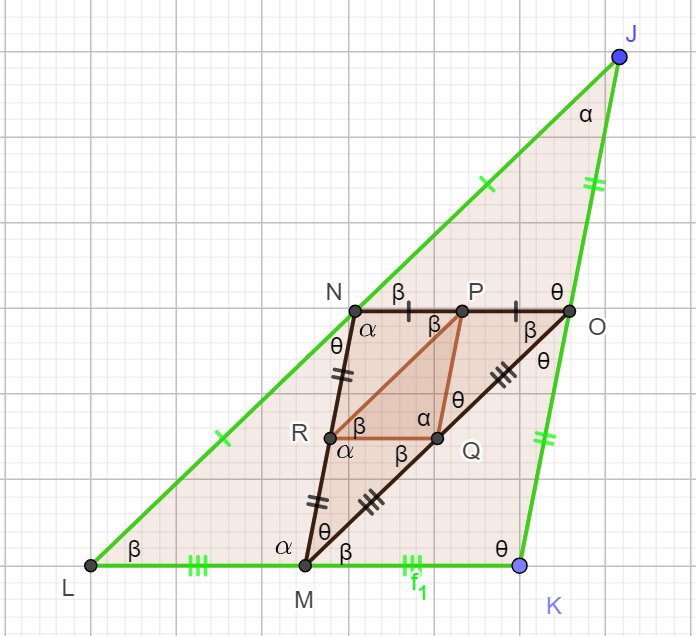
Points M, N, and O are the midpoints of sides KL, LJ, and JK, respectively, of triangle JKL. Points P, Q, and R are the midpoints of NO, OM, and MN, respectively. If the area of triangle PQR is 27, then what is the area of triangle LPQ?

Note:

The line joining the midpoints of a two sides of a triangle is parallel to the 3rd side. You can look up the proof if you wish.

So

KJ is parallel to MN is parallel to QP

ans

KL is parallel to ON is parallel to QR

So every triangle in this figure is similar to all the others.

LPQ=27u^2

So the area of MNO = 4*27 =  108u^2

And the area of LJK = 4*108 = 432u^2

Before I think on it any further can you confirm that it really is triangle LPQ that you want the area of ...

Here is the diagram.

y=(x+4)(x+1)(x-2)^2

If a normal distribution is graphed then the Highest column would be in the middle.

Then it would go down equally on both sides. It would look symmetrical about the middle.

If more of the scores are on the top side or on the bottom end then the graph/distribution is not normal,  it is skewed.

So is this distribution normal?

How many flavours are there?  Try proof reading your questions.

Draw a sketch.

You want the perpendicular distance from the chord to the circle. (Which will bisect the chord)

Use Pythatoras's theorem.

The question is not complete

Nice work asinus.  And guest too of course. :)

If I understand the question properly the team will consist of Grogg and Alex and 3 others from a group of 7

That will be  7C3 ways